This model illustrates the variation in cell distribution from that which would be expected by the division of flow at a microvascular bifurcation.

Description

This model represents the variation in hematocrit distribution that occurs at 
a microvascular bifurcation. With internal diameters larger than about 30 
microns the division of red blood cells is roughly proportional to the division 
of blood flow at a bifurcation. Below 30 microns, the cell-free layer that is 
present near the cell wall begins to affect the relationship between the division
of flow and cells. Pries, Ley, Claassen and Gaehtgens originally characterized 
this variation in their 1989 Microvascular Research paper (see References below). 
Drawing a parallel to the Fahraeus effect, which is the reduction of the supplied
hematocrit in narrow vessels, they called this the network Fahreaus effect. 
Refinements have been made to the model by Pries, Secomb, Gaehtgens and Gross in 
1990 and more recently by Pries and Secomb in 2005.

The result of the variation in hematocrit distribution can be illustrated by this
example from the JSim applet below. A 10um diameter parent vessel divides into 
an 8um and a 4um daughter vessel. The parent vessel has a discharge hematocrit of
0.45. If the division of flow is 80%/20% between the large and the small 
daughter vessel, from the graph in the JSim applet we can see that the division 
of cells is roughly 85%/15%. 
  

Equations

The hematocrits in the large and small branches in this example can be calculated as follows:

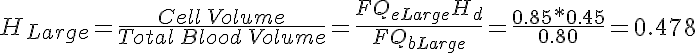
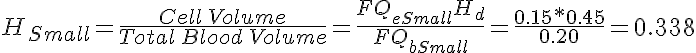
Therefore, a small variation in the division of cells at a bifurcation can lead to a wide heterogeneity in actual hematocrit values across a network.

The relationships for the phase separation effect as presented here reflect the most recent refinement made to the model by Pries and Secomb in 2005.

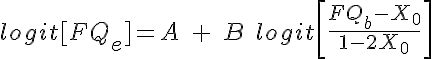
where FQ_e is the fractional erythrocyte flow in daughter branch 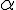 , A is the asymmetry parameter which strongly depends on the diameter ratio of the two daughter branches, B is the nonlinearity parameter which decreases with decreasing hematocrit, FQ_b is the fractional blood flow into the daughter branch  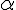and X₀ is the fractional blood flow value below which no cells enter the branch. The accompanying expressions for A, B and X₀ are:

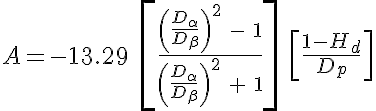
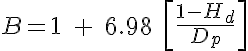
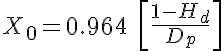
where 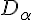 is the internal diameter of daughter branch 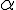,  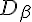is the internal diameter of daughter branch  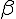and H_d is the discharge hematocrit of the parent vessel. Finally the definition of the logit function is given below:

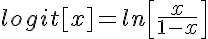
The equations for this model may be viewed by running the JSim model applet and clicking on the Source tab at the bottom left of JSim's Run Time graphical user interface. The equations are written in JSim's Mathematical Modeling Language (MML). See the Introduction to MML and the MML Reference Manual. Additional documentation for MML can be found by using the search option at the Physiome home page.